The Most Beautiful Theorem In Mathematics Euler S Identity By Ali However Mathematics Medium
Combines several of the most important mathematical constants e, π, i, and 1 Construct a formula which is equal to zero, using each of those constants once in your expression Hint remember that θ in eiθ is in radians We'll go straight to the answer eiπ 1 = 0It requires an Imaginary (1^5) and Complex plane of numbersbut is e^π pointing to something even more simple and sophisticated at the same time?
E^iπ+1=0 proof
E^iπ+1=0 proof-It's written both ways, because both equations are the same Some mathematicsfanatics enjoy writing e^i*pi 1 = 0 because it provides the fundamental numbers of math 0 and 1 are the beginning of the natural numbers, and pi and e represent the simplest, yet arguably the most important transcendentalWhat if we perhaps replace i (Root1) with the Fractal Root of 1?
The Most Beautiful Equation Of Math Euler S Identity Science4all
e iπ = cos π i sin π cos π = 1 and sin π = 0 Consequently, we arrive at an elegant and powerful result combining three of the most interesting variables in mathematics 'e', 'i' and 'π' e iπ = 1 This is more commonly written as e iπ 1 = 0 Is 'Euler's Identity' ((e^iπ)1 = 0) really the "Most Beautiful Equation in Mathematics"? The difference comes about because while taking a power produces one answer, but taking a root produces several kraguljacm says at 735 pm The exponential function over the complex field has period 2pi So e^ipi*e^ipi = e^ (2pi)i = e^0 = 1 More generally e^ (2npi)i = 1 for n = 1, 2, 3, Bob says
The proof there is very interesting as it illustrates the difficulty of the idea of representing a generic 0,b, where b is a positive constant aware of the "nerdy" formula eiπ 1 = 0 and how it is truly astounding that five special numbers appear in a simple formulaThe identity e^ (iπ)1 = 0 is a well known equation that can be proven mathematically It is an identify that contains the most beautiful entities encountered in math, namely π, i, e, 0 and 1E iπ = cos π i * sin π Then, since cos (π) = 1 and sin (π) = 0, e iπ = 1 and e iπ 1 = 0 The proof of Euler's Theorem involves the definition of e, by a Taylor's series expansion of ez (where z is a complex number), De Moivre's formula, and the
E^iπ+1=0 proofのギャラリー
各画像をクリックすると、ダウンロードまたは拡大表示できます
3 |  3 | 3 |
3 |  3 |  3 |
 3 | 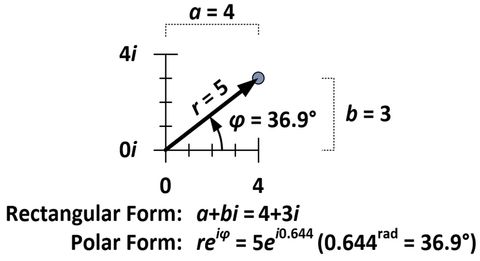3 |  3 |
 3 | 3 | 3 |
3 |  3 |  3 |
3 |  3 |  3 |
 3 |  3 | 3 |
 3 |  3 |  3 |
3 | 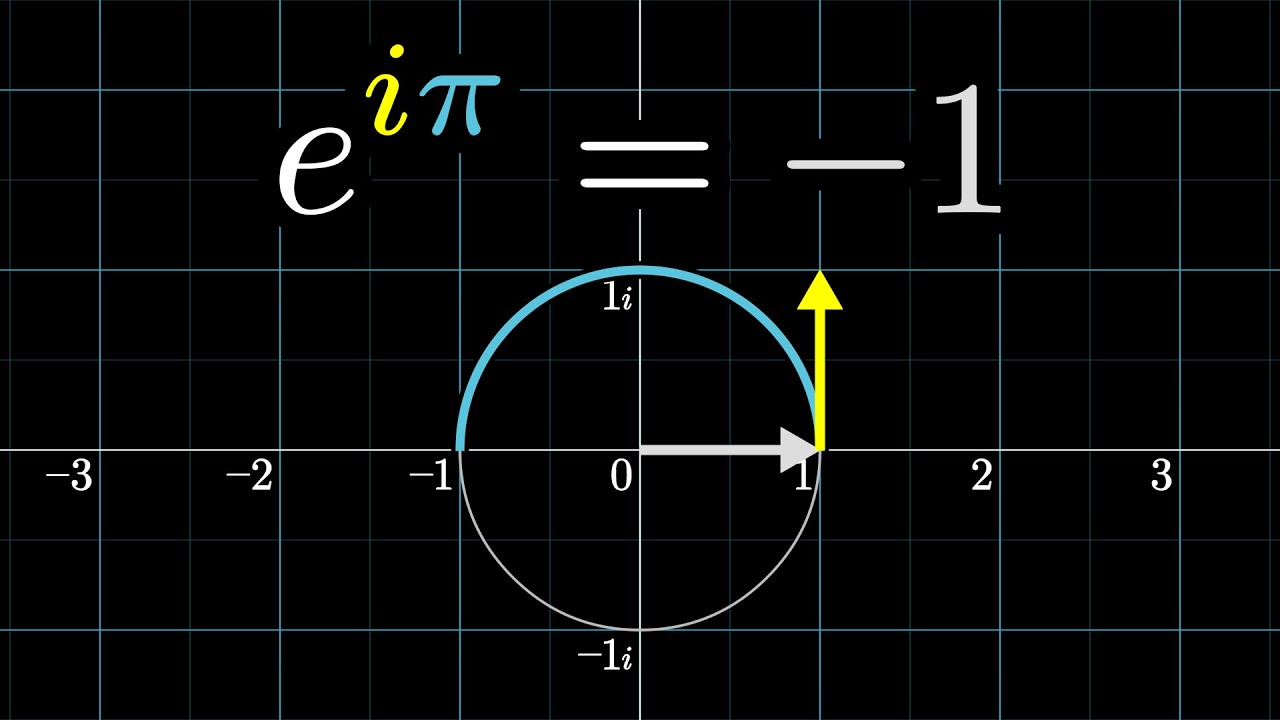 3 | 3 |
 3 | 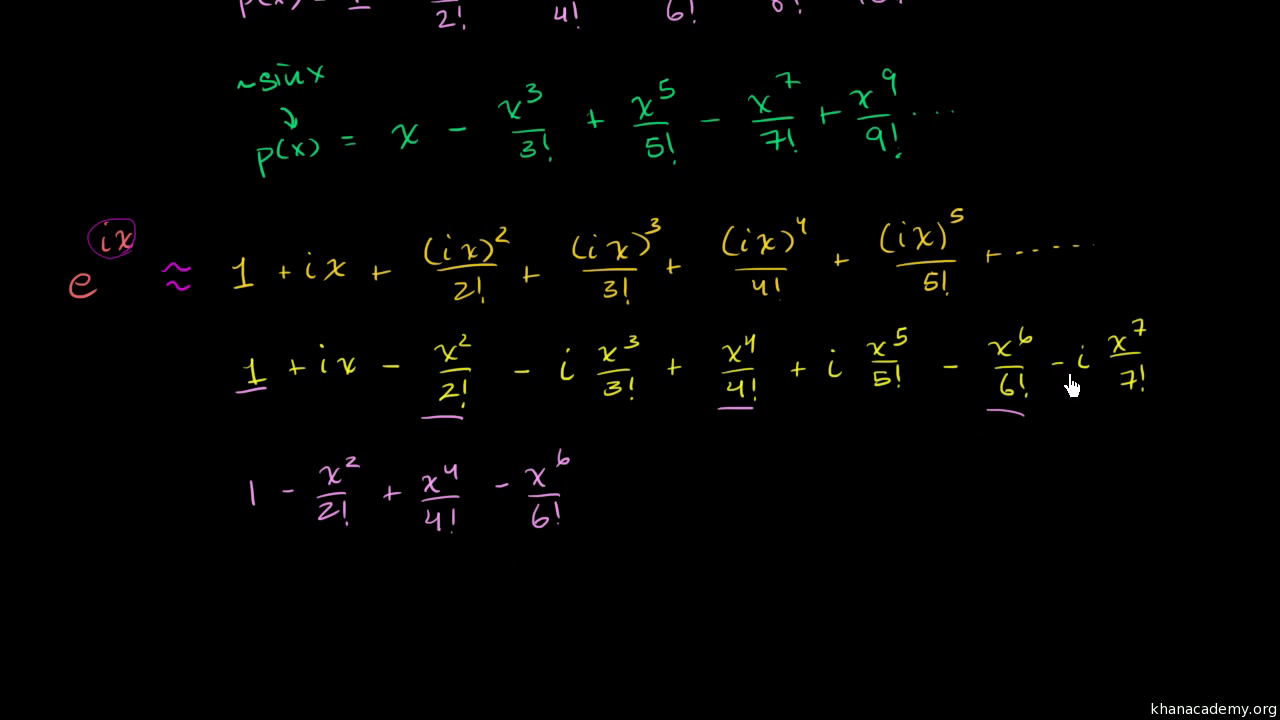 3 | 3 |
 3 | 3 |  3 |
 3 | 3 |  3 |
 3 | 3 |  3 |
3 |  3 |  3 |
 3 |  3 | 3 |
 3 |  3 | 3 |
 3 |  3 |  3 |
3 | 3 |  3 |
 3 |  3 |  3 |
 3 |  3 |  3 |
 3 | 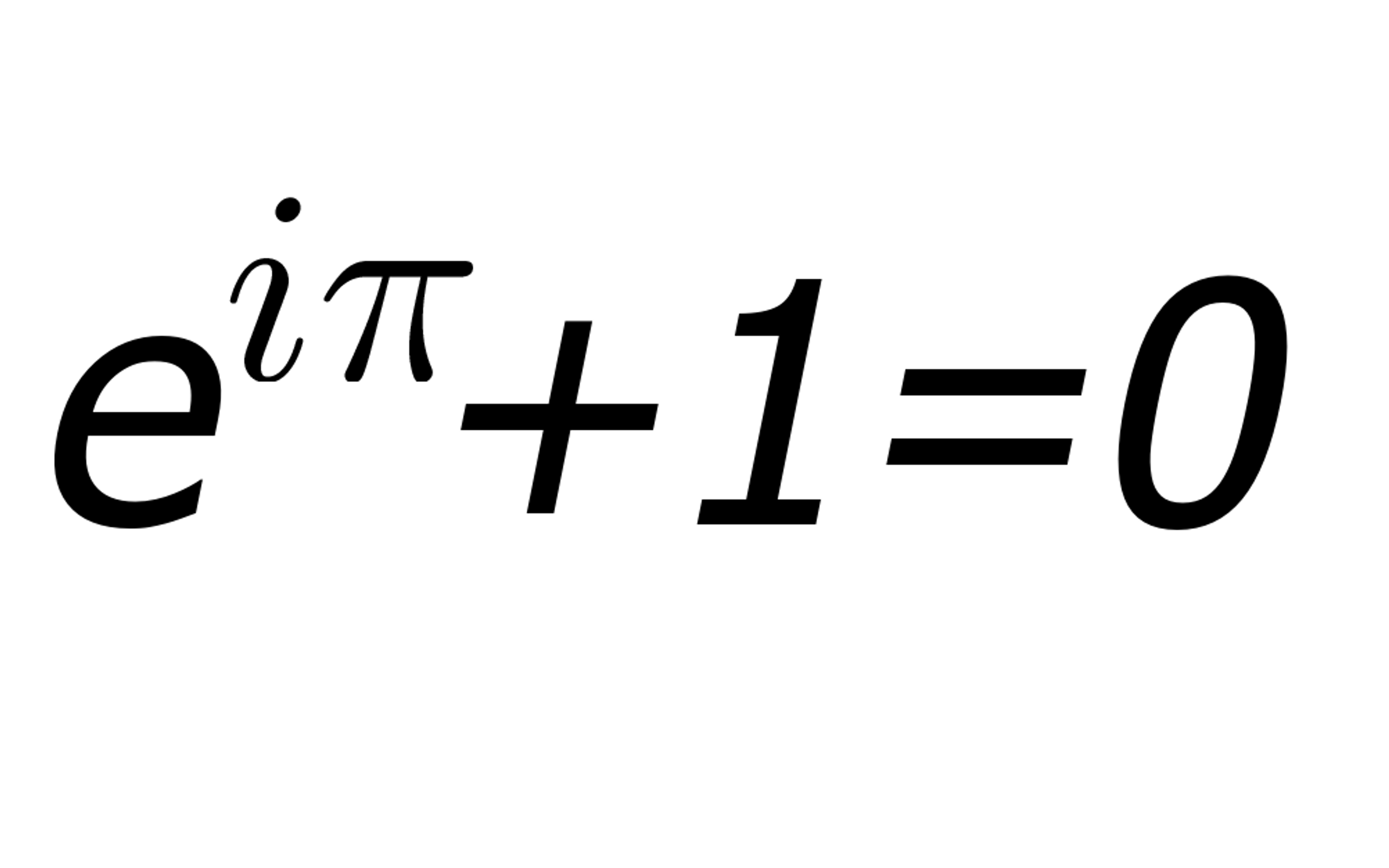 3 | 3 |
3 | 3 |  3 |
3 |  3 |  3 |
3 | 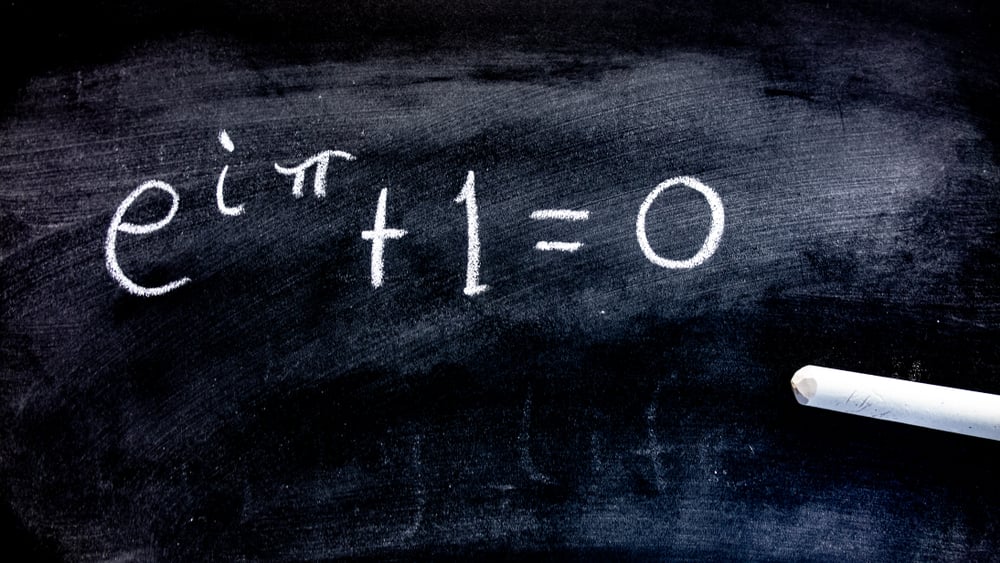3 | 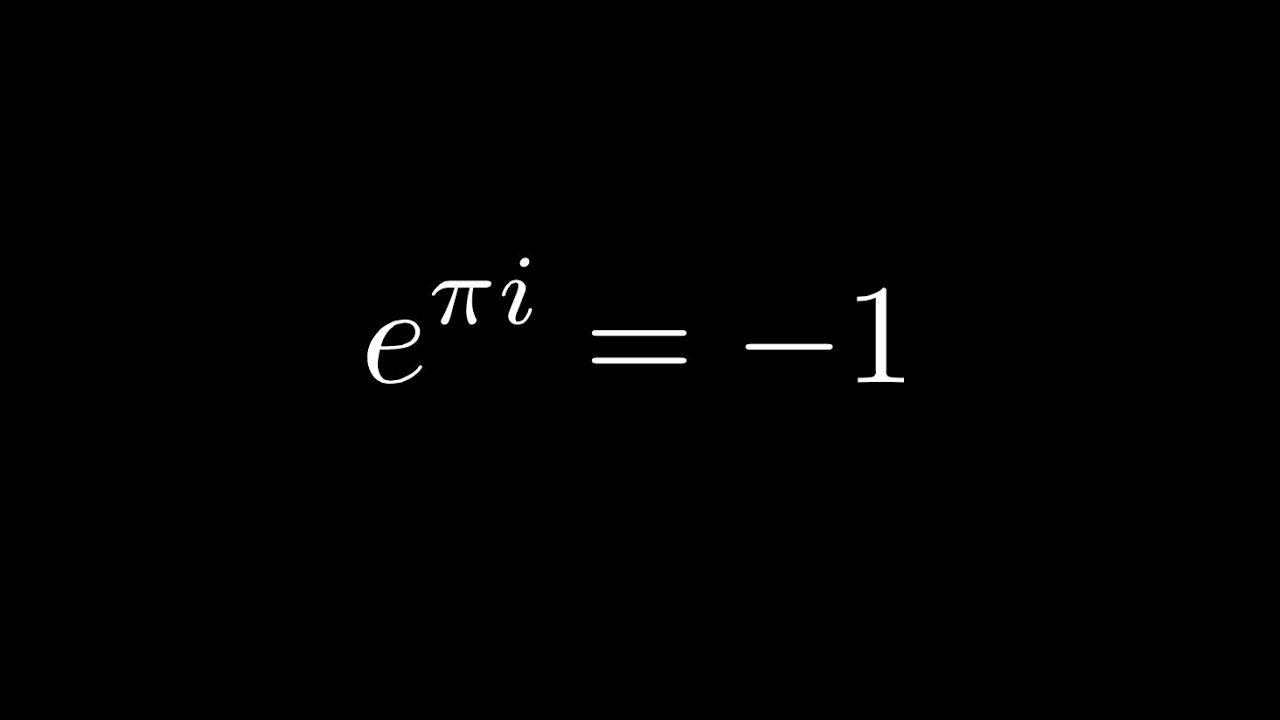 3 |
 3 |  3 |  3 |
 3 |  3 |  3 |
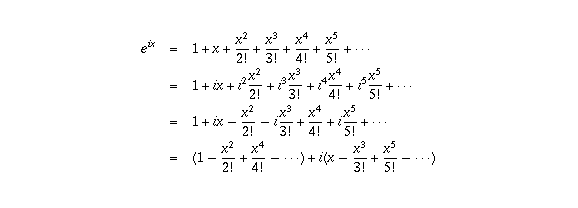 3 |  3 | 3 |
 3 |  3 | 3 |
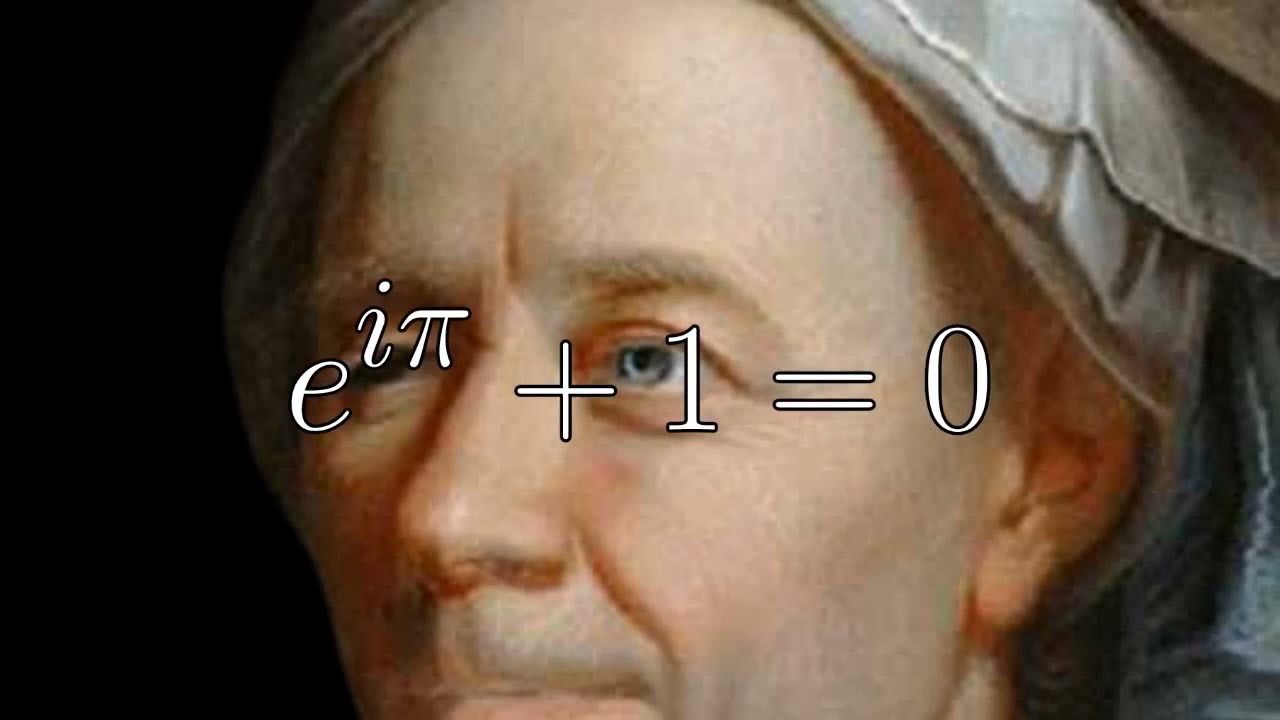3 | 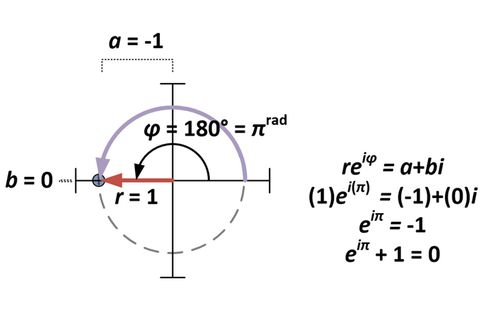 3 |  3 |
 3 | 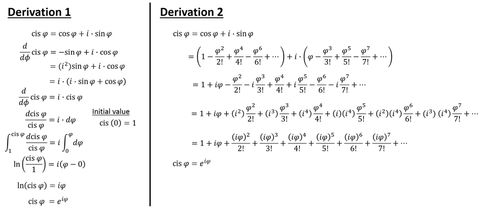 3 | 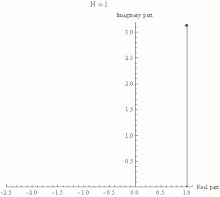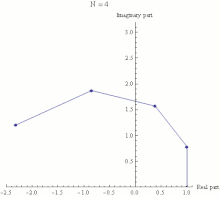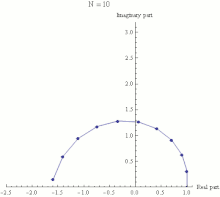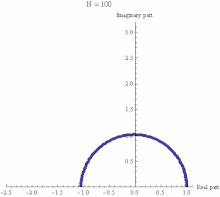 3 |
 3 |  3 |  3 |
 3 | 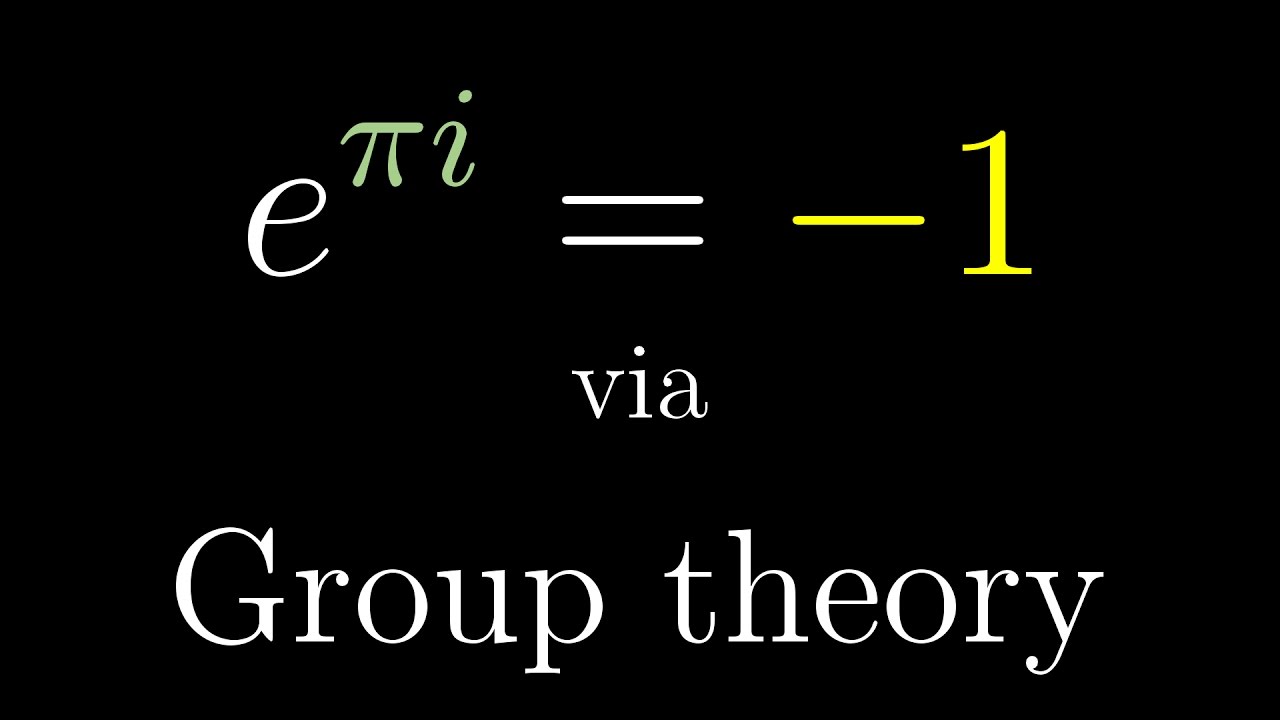 3 |  3 |
 3 | 3 |  3 |
 3 |  3 |  3 |
3 |  3 |
With the polar form of complex numbers established, the matter of Euler's Identity is merely a special case of abi for a = 1 and b = 0 Consequently for theWe can think of z 0 = abias a point in an Argand diagram but it can often be useful to think of it as a vector as well Adding z 0 to another complex number translates that number by the vector a b ¢That is the map z7→ zz 0 represents a translation aunits to the right and bunits up in the complex plane Note that the conjugate zof a point zis its mirror image in the real axis
Incoming Term: e^iπ+1=0 proof,
